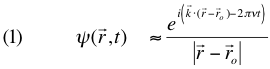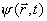
, called a probability amplitude, with any point 
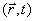
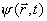
in space and time. contains part of the information encoded in the system's quantum state,
and allows one to compute the probability of the
possible outcomes of measurements involving kinematical variables such
as position and momentum. The square modulus of the wave function, 
, gives for example the probability of finding the system in 
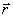
at time t (this important result can be derived from the Born rule). According to de Broglie's relations, the wave function associated with a beam of particles of equal momentum p and energy E, is a monochromatic wave of wavelength λ = h/p and frequency ν = E/h . For instance, a spherical wave provides a good approximation of the wave function associated with a particle propagating from a point-like source:
(
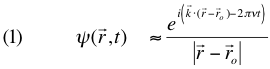
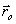
being the position of the source, 
being the wave vector governing the propagation of the wave, whose modulus is 2π/λ). Notice that, in this example, 
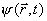
differs significantly from zero over a large region of space. This means that the distribution which describes the probability to detect the particle at a given location is not localized
. Well-localized systems (i.e.
corpuscular objects) are not associated with a monochromatic wave, but
rather with a wave packet. Wave packets can be regarded as superpositions of an infinite number of monochromatic waves of different wavelengths, interfering destructively anywhere but in a small region where they add up
constructively giving rise to a signal. The spectral and spatial
extensions of a wave packet have to obey the uncertainty relations. The interference pattern observed in the double-slit experiment is easily understood in terms of wave function. The two slits split the wave associated with the incoming atom beam into two secondary spherical waves
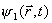
and 
(like in figure 3). The atom's wave function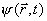 
after the double-slit filter is given by a weighted sum of these two
secondary waves. This is why an ensemble of atoms produces interference fringes on the detection plate: the maxima and minima of probability reflect the different phase relations existing between the two waves 
and 
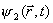
at different points of the detection screen (see figure 1 and the related discussion). In analogy with the optical case, the spacing between the fringes is proportional to the wavelength λ of the atoms. This explains why interference cannot be observed in a double-slit experiment with macroscopic bodies. Indeed, according to the de Broglie relations, the inter-fringes spacing turns out to be extremely small for large mass objects. So tiny, in fact, that no physical detector has enough resolution to distinguish the interference between maxima and minima.
In general, however, whether an ensemble of systems does or does not display interference is not a matter of size. Rather, it depends on the amount of information in principle available to the observer. To make this point clear, let's go back to the double-slit experiment and compute the probability p(x) of detecting an atom at a point of coordinate x along the axis perpendicular to the atom beam. Following an approach due to Richard Feynman, we consider the two possible ‘paths’ connecting the atom source to each point of the detection screen (see figure 6). We will call the paths coming from slit 1: ‘↑’ paths, and the paths coming from slit 2: ‘↓’ paths.
Figure 6. For each point of the detection screen one can imagine two converging paths for the atom, each one coming from one of the slits.
We can also associate a state vector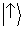
to the atoms following a ‘↑ path’: 
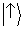
is the state vector that predicts for the atoms' position on the screen the distribution observed in an apparatus where only slit 1 is open (red curve of figure 4). Conversely, we call 
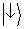
the state vector that predicts the distribution observed in an apparatus where only slit 2 is open (blue curve of figure 4). Having stated these definitions, we can discern two cases.
• Case 1 – INDISTINGUISHABLE PATHS
Suppose that there is no way to say whether the atom has followed a ‘↑’ path or a ‘↓’ path (the paths are said in this case to be indistinguishable). The state vector representing this situation is the following:
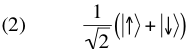
Applying Born’s rule to state (2), one can formally derive the spatial distribution p(x) expected in this case. Our previous discussion on wave functions provides us with the recipe necessary to carry out this simple calculation. We get:
and 
correspond to the red and blue distributions in figure 4 respectively. Besides the weighed sum of these two terms (green curve A in figure 4), the probability associated with state (2) contains ‘cross’ terms. It is easily seen using eq. (1) that cross terms oscillate as a function of x. When added to the first and second term, this oscillating function give rise to the typical interference pattern (green curve B in figure 4). • Case 2 – DISTINGUISHABLE PATHS
Let's consider now the case in which it is possible to discriminate the paths (without disturbing the motion of the atoms). Suppose for example that the atoms are excited and decay emitting a photon exactly while passing through the slit (figure 5). Detecting the emitted photon would bring the ‘which slit’ information to our knowledge. Therefore, ‘↑’ paths and ‘↓’ paths are now distinguishable (in the sense that they can – in principle – be distinguished). Let W be the photon observable carrying the ‘which slit’ information, and let's conventionally call ‘
’ the outcome of W that indicates slit 1 and ‘
’ the outcome of W that indicates slit 2. The state vector corresponding to the new experimental configuration is the following entangled state, involving the atom and the emitted photon: 
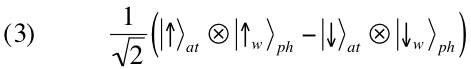
According to the definition of entangled state, if the photon indicates
, then the atom will follow the probability distributions predicted by state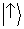 
, whereas if the photon indicates 
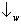
, then the atom will follow the probability distributions predicted by 
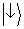
. Since each photon indicates either 
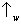
or 
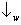
, it's clear that each atom is compelled to follow either the spatial distribution associated to 
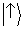or the one associated to 
. Therefore, if we look at the detected atoms disregarding the information supplied by the photon, we will see a spatial distribution that is simply the weighted sum of the probability distributions predicted by 
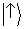
and 
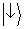
respectively. The weights of the sum are the probabilities for the outcomes 
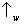
and 
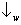
to occur, namely ½ and ½. 
Cross terms, and hence interference fringes, are absent in this case (compare to the discussion on the entangled states of spin ½ particle).
The disappearance of interference when the ‘which slit’ information becomes in principle available should not appear too surprising. The atom state vectors
and 
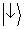
can be expressed as superpositions of either position eigenstates or momentum eigenstates. Therefore, they assign probabilities not only to different positions, but also to different momenta. In particular, state 
assigns probability 0 to momenta indicating that ‘the atom is coming from slit 2’, whereas the opposite is true for 
.
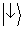
Thus , making the ‘which slit’ information available amounts to
(partially) measuring the atoms' momentum. Position and momentum being incompatible observables, a measurement of momentum unavoidably modifies the spatial distribution of the atoms in any subsequent measurement of position. Interference, in particular, is washed out (see the discussion of quantum states for more details). Feynman's rules are a powerful tool that can be used in several contexts, once a set of ‘paths’ and the corresponding probability amplitudes have been recognized. Interference itself is a general feature of quantum phenomena, not limited to position measurements. Regardless of the way a system is prepared, one can find an observable such that the probability distribution associated with its outcomes displays interference (meaning that some outcomes are found with high probability, while others are never found). In general, if the state of the system can be written as a superposition of the eigenstates of an observable A, interference fringes are observed when measuring an observable B incompatible with A. The condition for interference to be observed is that there be no ‘which path’ information available (neither in principle), i.e. that there is no observable C whose outcomes are correlated with those of the ‘path observable’ A (see the example of spin observables).
Interference effects illustrate the deep connection between the principle of superposition, incompatible observables and complementarity. This link shows in turn to what extent the mathematical core of quantum theory is determined by the relations existing among the observables we use to ‘structure’ physical phenomena (see implications).