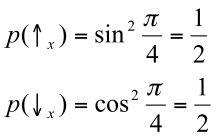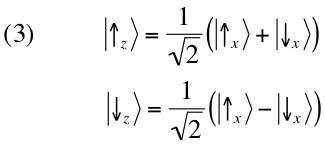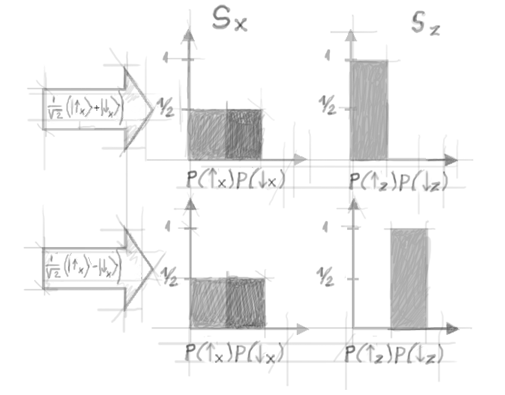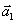
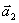
SUPERPOSITIONS > Origins Quantum states can be represented by vectors belonging to a so-called Hilbert space. Vectors provide an ideal framework to represent statistical regularities, especially when incompatible observables are involved. To see how this works, let be a vector of unit length in the plane and consider its projections on two orthogonal axes and (figure 5). By Pythagoras' theorem:



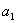
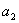
Now suppose that we measure an observable A that can only take two values, and . The probabilities of getting and vary depending on the way the measured system has been prepared.

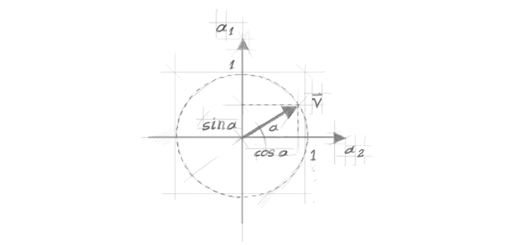
Figure 5. A unit vector in the plane and its projections on the axes.

Nevertheless, since and represent mutually exclusive outcomes exhausting the spectrum of the possible occurrences, we know a priori that, in any case, the corresponding probabilities meet the condition:



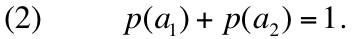
The predictive formalism of quantum theory is based on the parallelism between eq. (1) and (2). It is summarized by the following rules:

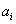
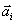
I. The values that an observable A can take are represented by orthogonal axes spanning a state space.

II. Each experimental procedure which ‘prepares’ a system before a measurement of A is represented by a state vector 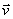belonging to the state space and having unit length.

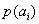
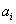
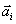
III. (Born rule) If we measure the observable A, the probability to get the value is given by the square modulus of the projection of onto the axis:

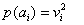
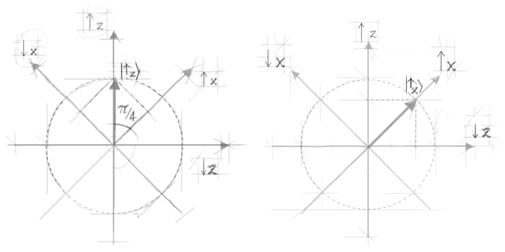
Figure 6a and b. Representation of the 'up' eigenvector of Sz . The antiparallel vector would do the job as well - we arbitrarily choose vectors belonging to the positive unit semicircle to represent quantum states.

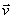
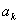
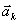
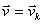
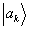
An important corollary of rules I-III is the following: the state vector which corresponds to the special case in which we can predict with certainty (i.e. with probability 1) that the measurement of A will yield the value is the unit vector lying on the axis ( ). Following Dirac's notation, this vector is denoted and is called an eigenstate (or eigenvector) of A.

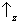
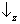
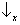
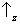
Let's apply these simple rules to the Stern-Gerlach experiments discussed in the section on experimental evidence. We are concerned with two spin observables: S_z, which can take the two ‘values’ and ; and S_x, which can take the two ‘values’ and . In the first step of the experiment (figure 2) only those atoms are selected for which a measurement of S_z yields . 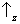For these atoms, we are certain to find if the measurement of S_z is repeated. Therefore, the associated state vector is . 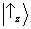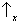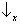If, on the sample of atoms thus prepared, we now measure S_x, we find for half of the atoms and for the other half (step 2 of figure 3). Can this result be represented in our state space? 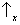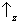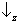Yes, it is sufficient to take the two axes corresponding to and (see rule I) as forming an angle of π/4 with the axes corresponding to and (figure 6a). 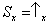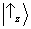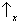According to rule III, in order to find out the probability of finding we have to project the state vector onto the axis corresponding to (figure 6a). In the same way we compute the probability that the measurement of S_x will yield . 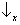We get:



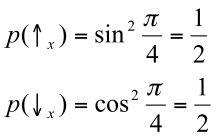
The result of the third experiment (figure 3) can be straightforwardly formalized in the same way, observing that the first two apparatus ‘prepare’ the state 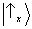(figure 6b).

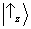
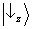
Let's see how interference phenomena are taken into account by this simple model. Exploiting the elementary rules for the composition of vectors in the plane, the vectors and can be rewritten as follows:



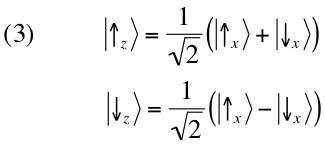
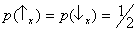
Notice that both these states predict the same probability distribution for a measurement of S_x: . Therefore, the two states (or, to be more precise, the corresponding preparations) cannot be distinguished a posteriori by performing such a measurement. The two preparations yield nonetheless opposite statistical results if a measurement of S_z is performed (figure 7). In the former case one always finds ‘ ’; whereas in the latter, this never happens. This ‘never’ is the signature of interference (compare to the bright and dark fringes of the double-slit experiment). Notice that the only mathematical difference between the two states (3) is the sign of the superposition: plus in the former case, minus in the latter. 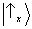This sign expresses the phase relation existing between and . 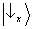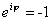(Using complex notation, or depending on whether φ = 0 or φ = π; it is worth making a comparison with waves.)

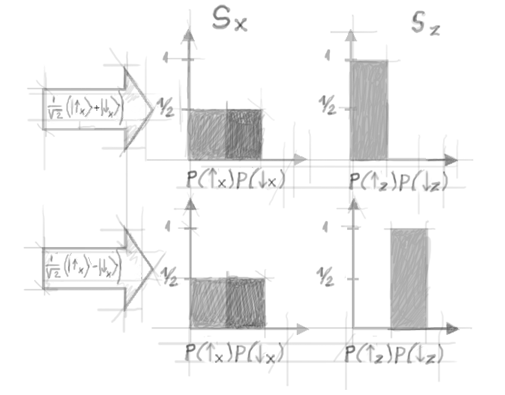
Figure 7. Comparison of the probability distributions predicted by two different superpositions of Sx' eigenstates.

So far, We have been concerned with a special class of observables, namely those observables that can be represented by different orthogonal frames within the same state space. The probability distributions associated with such observables are rigidly connected to one another. In the case of S_z and S_x, for instance, we have seen that, given an equiprobable distribution of ‘up’ and ‘down’ for S_x, the statistics of S_z necessarily follows one of the two distributions represented in figure 7.

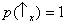
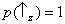
However, there exist observables whose respective probability distributions are a priori completely independent. A typical example is provided by the spin observables of two distinct particles. Particle 1 can be prepared in such a way that and particle 2 in such a way that . (This cannot be done if S_x and S_z are measured on the same particle, since S_x and S_z are incompatible observables.) In general, however, once two quantum systems have interacted, they become correlated in such a way that measurement results can no longer be predicted by just assigning an individual state vector to each partner system. Instead, a unique, global state vector has to be associated with the whole pair. This state is constructed by combining the individual state vectors, but belongs to a larger state space. An entangled state of two spin ½ particles is the following:



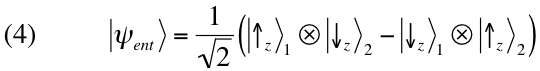
The statistical predictions associated with are very important. The probability of finding ‘up’ or ‘down’ if S_z (or any other spin component) is measured on each particle separately is 1/2. Furthermore, if one of the particles is found to have spin ‘up’ in the z direction, the result of any spin measurement performed subsequently on the other particle will follow the statistics predicted by . If, conversely, the first particle is found to have spin ‘down’ in the z direction, then the statistics of the other particle will be that corresponding to . 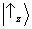Due to the peculiar form of state (4), analogous correlations exist for any spin component. 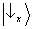Thus, if, for example, particle 1 is found to have spin ‘up’ in the x direction, particle 2 will follow the predictions of ; and so on (the situation is represented in figure 4). 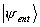Indeed, preserves its mathematical form if expressed in terms of the eigenvectors of S_x:

.

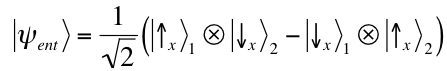
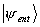
The probability distributions observed in measurements performed on a single particle are fundamentally different depending on whether the particle has or has not an entangled partner. In the former case, as shown by figure 8, interference effects are washed out (this can readily be derived from the above definition of by using formulas (3); see also complementarity).

In the toy-model used for our presentation, the state space was the real plane of Euclidean geometry and the outcomes were represented by axes. However, a complete analysis of all possible Stern-Gerlach measurements shows that this space is insufficient to provide a complete representation. A richer geometrical structure, the Hilbert space, defined on the field of complex number, must be introduced. Within this structure the outcomes are represented by subspaces, and the observables by combinations of projectors over these subspaces.

Figure 8. Probability distributions encountered when the tested particle (particle 1) has an entangled partner.