
Figure 1. After having undergone radioactive decay, an atom is lighter.
In order to test eq. (1), Einstein proposed to ‘weigh’ an atom before and after it had undergone radioactive decay. The implementation of such a proposal, however, was technically difficult. The first tests used a different method, involving particle collisions. When a lithium atom (Li) is bombarded with a proton (p), two α-particles emerge. On the one hand, a decrease in the total rest-mass is observed in this process: the rest-mass of the ensemble proton + lithium atom is greater than the total rest-mass of the two α-particles (which can be determined independently). This is at odds with the classical principle of mass conservation.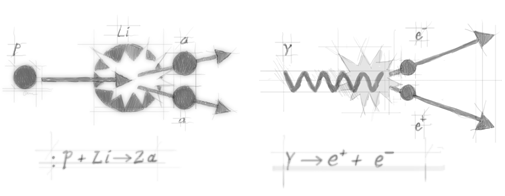
Figure 2. Two reactions observed in particle physics:
On the other hand, there is an increase in the total kinetic energy: the kinetic energy of the proton is less than the total kinetic energy of the two α-particles. (The lithium atom as a whole can be considered to be at rest and does not contribute to the balance.) This is at odds with the classical principle of energy conservation.In another experiment, an electron-positron pair (whose rest mass is different from zero) emerges from a γ ray photon (whose rest mass is zero). This is an example of how pure electromagnetic energy is converted into massive particles. Interestingly, for the conversion to take place, the γ rays frequency must overwhelm a threshold value. To understand this fact, we first observe that the energy of photons depends on their frequency according to the relation E=hν (see complementarity). Given eq. (1) and assuming that the total mass-energy involved in the process is conserved, this means that the energy of the γ photons must be equivalent at least to the sum of the rest masses of the electron and the positron. (The surplus of electromagnetic energy provides the electron-positron pair with kinetic energy, i.e., with a non-zero velocity).